Part III Lecture Notes Part III Seminar Series Physics 7 Series

Part III Seminar Series

Part III of the Mathematical Tripos, University of Cambridge (November 29, 2018 and March 14, 2019).

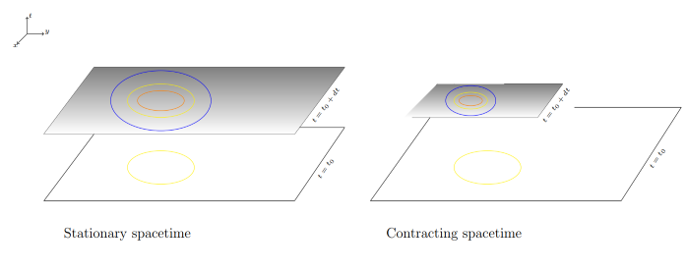
A comparison of light rays in stationary and contracting spacetimes. In black hole spacetimes, there may exist "trapped surfaces" on which future-directed light rays are generically forced to close up in the future. The Penrose singularity theorem establishes one set of sufficient conditions to prove that spacetimes with closed trapped surfaces are singular. Image credit: [arXiv:1410.5226].

To supplement my coursework at the University of Cambridge, I prepared two presentations for the Part III Seminar Series, in which various students chose topics related to lectures or (mathematically-oriented) personal interests and presented to the other students in their cohort. In preparation for my essay on Penrose's strong cosmic censorship conjecture, I opted to present on the nature of singularities in general relativity (for the Michaelmas seminar series) and the quasinormal mode solutions to the wave equation in curved spacetime (for Lent).

I gave the Michaelmas term talk as a 20-minute PowerPoint presentation, and the Lent term talk as a 30-minute chalkboard lecture. I also prepared a five-page typeset handout to accompany my lecture content for Lent, some of which later made it into my essay.

A Survey of Singularities

In the study of classical gravity, singularities often emerge when one tries to describe even the simplest spacetimes. However, it also seems to be true that in many cases, these singular points or surfaces are somehow hidden away from physical observers, e.g. by event horizons or intrinsic instabilities. This observation led Roger Penrose to conjecture that under certain conditions, singularities are always hidden away behind event horizons, a conjecture that remains unresolved to this day. In this talk, I discussed some of the difficulties in precisely defining what a singularity is in general relativity, and reviewed arguments from Geroch, Hawking, and Ellis to motivate the notion of incomplete geodesics as a sufficient criterion for a spacetime to be singular.

I outlined some of the main theorems regarding singularities in general relativity (due mainly to Penrose and Hawking), and stated some of the broader impacts of these theorems. I also described two conjectures about singularities regarding Penrose's idea of "cosmic censorship" and gave a preliminary summary of some recent work on a proposed violation of the strong cosmic censorship conjecture.

Quasinormal modes in the eikonal limit

As solutions to a modified wave equation in curved spacetime, quasinormal modes are a key tool for studying perturbations of black hole spacetimes. They are especially useful in understanding the stability of horizons and the formation of singularities after perturbation. In this talk, I described the geometric optics ("eikonal") limit for the calculation of quasinormal mode frequencies, in which the angular oscillations of these modes are significant. I also discussed the use of quasinormal modes in addressing one of the major outstanding problems of mathematical relativity, the strong cosmic censorship conjecture.

I presented a simplified derivation of the quasinormal mode frequencies in this limit by considering the case of spherical symmetry. I then demonstrated that the quantities which appear in the final expression for these frequencies have a simple physical interpretation, as these modes are closely related to unstable photon orbits around the black hole.

Documentation